TASK ONE
When you click on one of the photo options below, a bubble chamber photograph will appear. For each one of these, some of the particle collisions and decays are labelled with a letter. You are given a list of various types of collisions and decays and asked to identify each of these with a particular location (letter) on each of the photographs. In some cases you may also be able to identify some of the particles produced in the collision using the ideas discussed in the introduction.
Once you have selected a photo, use the two scroll bars to see all of it. Then answer the questions.
TASK TWO
Some more detailed questions have been set on two of the photograhps above.
When you have tried TASK ONE, you might like to try these.
This article was written by Peter Watkins of Birmingham University. The web pages were produced by Tom Adams of Cheltenham College. Please e-mail your comments to them.
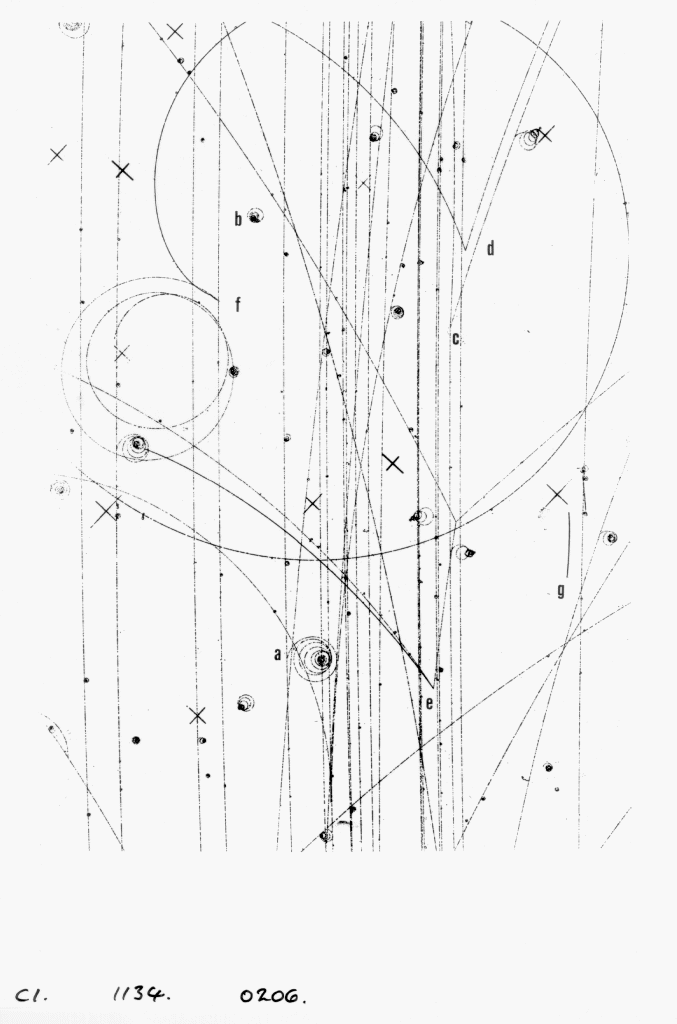
More detailed questions
- Why does the radius of the spiral get smaller?
- By considering the momentum of the particles before and after the collision say why there must be at least one other particle produced at c. What can you say about the charge of this particle?
- For the two particles produced in the decay at d use the radius of curvature to determine which has the largest momentum. Use your knowledge of momentum conservation to estimate the original direction of the neutral particle that decays at d.
- Consider the two particles that move to the left from e. How can you tell that they have approximately the same momentum? The lower track is much denser than the other one. What does this tell us about the relative mass of the two particles?
Click for the answers to these.
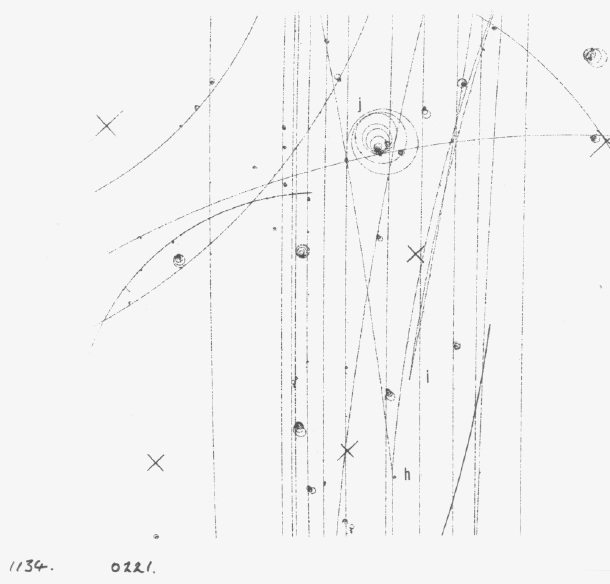
Photo 0221
- The negatively charged beam kaon interacts with the positively charged proton at h to produce a positive, negative and a neutral particle. The magnetic field is into the paper. Which of the particles produced at h is positively charged?
- Which particle has the highest momentum?
- Assume that all the particles in the interaction travel at approximately the speed of light and that the picture is full scale. Estimate the lifetime of the neutral particle produced at h which decays at i.
- Does this time indicate that the decay proceeds by the weak or strong force?
- How could you check if any neutral particles were produced at interaction h?
- If a single neutral particle were produced and this did not decay in the bubble chamber, how could its mass be estimated?
- How can the direction of the neutral particle which decays at i be estimated?
- How can the mass of this neutral particle be calculated?
Click for the answers to these.
TASK TWO ANSWERS 0206
- The electron experiences an electric force due to nearby atoms when it passes through the hydrogen. As its mass is much smaller than the proton the acceleration produced by this force is very large. Any accelerated charge loses energy by emitting photons and this means the electron loses energy and momentum rapidly which leads to a smaller radius of curvature because
| B q v = m v²/r
| r = m v/B q = p/B q | (where p is momentum)
| |
- Momentum conservation requires that at least one additional neutral particle must be produced and emitted to the left of the observed particles. The additional particle must be neutral as it leaves no track.
- The particle moving to the right has the highest momentum. The direction of the neutral particle can be estimated if it decays to the two observed particles and no additional neutral particle. The momentum of each of the two decays products is proportional to their radius of curvature. The momentum of the parent is the vector sum of the two decay particle momenta. The line of flight will always be between the decay products and in this case will lie closer to the line of the higher momentum negative track which is on the right. This means that the neutral particle is probably produced at the nearby kaon proton interaction labelled c.
- They have approximately the same curvature. The number of bubbles/cm is inversely proportional to v2 where v is the velocity of the particle. Where two particles have similar momentum e.g. the two nearby particles in this interaction then the velocity of the particle will be inversely proportional to its mass. The proton is the heaviest stable charged particle and has a mass around seven times that of a pion and so the bubble density will be around fifty times larger for the proton. This means that very dark tracks are usually protons. There are a few exceptions e.g. where a track is travelling towards or away from the camera but these can be eliminated when using photographs from three different cameras.
Return to the QUESTIONS or to the MAIN MENU
TASK TWO ANSWERS 0221
- The particle moving to the left is positive. Notice that it is the direction of curvature not motion that depends on the charge.
- It also has the higher radius of curvature and so it has the higher momentum.
- The neutral particle travels about 3.5 cms in the chamber before it decays. If it travelled at the speed of light then the time for it to decay is
t = 0.035/3 x 108 = 1.2 x 10-10 seconds
- Decays via the strong force occur in typically 10-23 seconds and so the weak force is responsible for the decay. The neutral particle is a Ko and as this is the lightest strange particle it does not conserve strangeness in its decay to two pions. As strangeness is conserved by the strong force the decay can only proceed by the weak force.
- In the A level Particle Physics topic SI units are always used. For some of the calculations described here it is much more convenient to use the units which are used in Particle Physics research. These have been introduced to avoid the need to have the speed of light (c) cropping up in all the equations. The commonly used energy (E) unit is GeV which is 109 electron volts. The units used for momentum (P) and mass (M) are GeV/c and GeV/c2 respectively and with these units the relativistic equation can be written as
Although this seems confusing it means that with these units that energy, momentum and mass always have the same dimensions.
One could check whether energy and momentum conservation is consistent with the observed particles. The momentum (PK) and rest mass (MK) of the incident kaon is known and so the kaon energy (Ek) can be calculated using
The target proton has zero momentum and mass MP and so has energy EP = MP and so the total initial energy E and momentum P can be computed using
The momentum P1 and P2 of each of the two charged outgoing particles can be measured from their radius of curvature using P = Bqr. Note the magnitude of the vector momentum, in the plane of the photograph, is obtained from this equation and then we need to measure the angle of the trajectory at the interaction point to convert to a vector momentum. We would need to use the photograph from all three cameras to measure these angles in three dimensions. If we could identify the outgoing particle types from the bubble density or other characteristics then we could use E2 = p2 + m2 to obtain the energy of each track. We can then compare the initial and final energy and momentum sums for the event to see if they are consistent allowing for the experimental errors on the measurements.
- If a single neutral particle were produced then its energy and momentum could be deduced from the imbalances discussed above. The mass can be evaluated using
- In this neutral decay the two charged decay tracks originate from one point and cross at a separate point in the chamber. Momentum conservation means that the neutral particle flight path follows the line joining these two crossing points. In this case it is clear that the neutral particle is produced at the interaction labelled h.
- If the neutral particle decays into the two observed charged particles then its mass can be estimated by the following method. If the identity of the two decay particles are known and their momenta measured then we can use energy and momentum conservation in the decay to evaluate the energy and momentum of the decaying neutral particle. Then its mass can be evaluated using
Return to the QUESTIONS or to the MAIN MENU